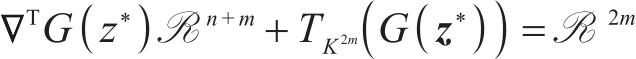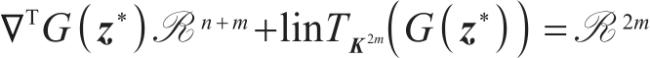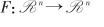 为单调的向量值映射;
为单调的向量值映射;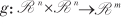 为连续可微映射; 为欧氏内积; ,且 , , ,每个 都是 维的二阶锥,集合 为一闭凸集。双约束条件是形如 的约束,该条件与经典问题的不同主要在于它将问题的参量与其自身变量联系在一起,这给双约束优化问题的研究带来了难度和挑战。
为连续可微映射; 为欧氏内积; ,且 , , ,每个 都是 维的二阶锥,集合 为一闭凸集。双约束条件是形如 的约束,该条件与经典问题的不同主要在于它将问题的参量与其自身变量联系在一起,这给双约束优化问题的研究带来了难度和挑战。1 预备知识
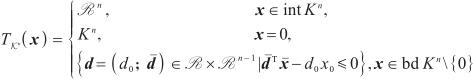
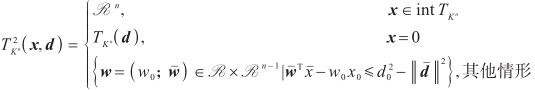
2 双约束二阶锥变分不等式的等价转换
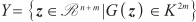 ,其中参量 ,约束条件为 ,证毕。
,其中参量 ,约束条件为 ,证毕。3 一阶必要性条件
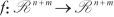 是一连续可微函数,约束条件中的
是一连续可微函数,约束条件中的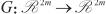 是一连续可微的向量值函数,
是一连续可微的向量值函数, 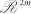 为一闭凸锥。
为一闭凸锥。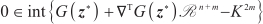
4 二阶充分性条件
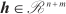 ,借助引理1中的公式,可推出如
,借助引理1中的公式,可推出如

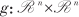
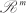 在
在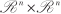 上是对称的,若有
上是对称的,若有
 关于变量
关于变量